Figures 1 and 2 show these same data in order to have a more visual feeling for the diameter changes. One set of straight lines connects the data points for each string, while the single straight line through the group of points representing each string are linear regressions estimated by the Excel algorithm for that data grouping.

Figure 1, giving data for the unison pair of 440 Hz A strings

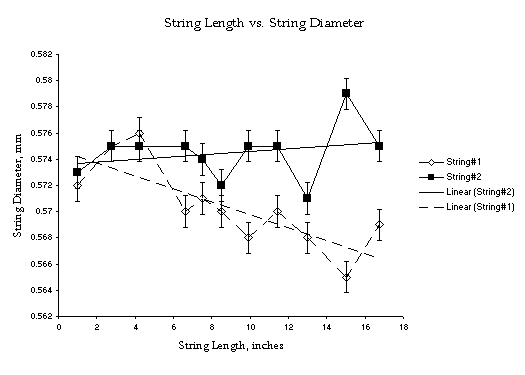
Figure 2, giving data for the unison pair of 329 Hz E strings

It’s clear that the string diameters are not constant, nor are any of the trends horizontal as they should be. But the actual changes involved seemed small to me and I wondered if I could calculate what the effects of these varying difference trends on the string frequency of fretted notes might be.

I began with the string formula:

f = (2L)^-1 * (T/m)^½

where f is frequency in Hz, L the string length, T the tension, and m the mass of the string per unit length. Since my goal was to compare the upper and lower halves of the string to one another in order to determine the effect of diameters on string frequency, I rearranged (1) to solve for T:

T = m * (f *2L)²

Since the tension for both halves of the string is the same, we can set the right hand side of the equation for each half of the string equal to the other:

m₁* (f₁ *2L)² = m₂ * (f₂ *2L)²

where m₁, f₁ refer to the upper portion of the string near the headstock, and m₂, f₂ refer to the lower half. The "2L" terms on both sides cancel. We rearrange once more and have:

(m₁/m₂) = (f₂/f₁)²

But we measured diameters not mass per unit length. So now what? We can obtain mass by dividing density by the unit length volume. And we obtain the unit length volume by using the formula for the volume of a cylinder:

V = pi * h * r ²

Where pi is 3.141728..., h the unit length and r the radius of the string. And since r = ½ * d, the diameter, then we have:

(m₁/m₂) = (dens. * pi * h₁ * .5 * d₁² )/ (dens. * pi * h₂ * .5 * d₂² )

The density, pi, h and 0.5 terms cancel so now we have

(m₁/m₂) = (d₁/d₂) ²

So now we can look at our fourth equation in terms of string diameters instead of string mass per unit length:

(d₁/d₂)² = (f₂/f₁) ²

The square terms cancel and we have the remarkably simple result:

(d₁/d₂) = (f₂/f₁)

That is, the ratio of the average "upper half" string diameter to the average "lower half" string diameter gives the ratio of the "lower half" string frequency to the "upper half" string frequency.

Since the customer worried about intonation near the mid string point, let’s look at string frequencies for the A and E strings in that region. The A frequency will be set at 880 Hz and the E frequency at 660 Hz for calculation purposes. Since most frequency meters give readings in cents or hundredths of an interval we need to go from Hz to cents in these regions. A semitone interval in the 880 Hz region is ~50 Hz and that in the 660 region is 38 Hz. So there are (100/50) 2 cents/Hz in the 880 Hz region and (100/38) 2.63 cents/Hz in the 660 Hz region.

The order of operations is as follows:

1. Calculate the average diameter of the string in each half of the string by summing the measurements for that half and dividing by the number of measurements.

2. Divide the upper string half average by the lower string half average to obtain the ratio of the lower string half frequency to the upper string half frequency.

3. Multiply the ratio times the string midpoint frequency and then subtract the midpoint frequency from the result.

4. Multiply the answer in 3. by the cents/Hz ratio for the frequency range in question.

Table 2 gives calculation results.

Table 2. Calculation results for frequency differences

How do we interpret the results of the last row of figures? First of all, we need to think of the ideal case, where the diameter of the string is constant throughout. In this case, the frequency ratio in row three of Table 2 is unity, and if the saddle is in the right place, the 12^th fret harmonic and 12^th fret fretted note will be the same. Of the four strings in Table 2, string# 2 comes closest to this situation.

But if the average diameter of the lower half of the string is smaller than the upper half as in string#1, then the notes of string#1 will be progressively sharper relative to string# 2. Why? The string formula (equation 1) shows us that mass per unit length is inversely proportional to frequency. That is, as string mass per unit length decreases (at constant tension and string length) frequency increases. Since mass per unit length and string diameter are both directly proportional to one another (at constant density), a smaller diameter equates to a higher frequency. As you move up the fretboard toward higher notes, the active string average diameter of string# 1 decreases both compared with its own upper half as well as the active string average diameter of string# 2. The notes of string#1 play sharper and sharper relative to string#2.

The case of string# 3 and string#4 is a little more subtle but equally irksome. The diameter trends of both strings are roughly parallel and not greatly disparate, so both will sound similar to one another as you move up the fretboard, but both will also will play progressively flatter because of the increasing average active string length diameter. Even if the saddle is in the correct position for a string of constant diameter, the fretted 12^th fret note will be flat ~5 cents compared to the 12^th fret harmonic.

What to do? I admit to being in a quandary. My customers and I really like the sound of the Savarez Alliance strings, but it’s clear that quality control of string diameter is a serious issue for the player with a keener ear. The Savarez Alliance strings are not trued (ground to constant diameter); I’m now on the lookout for strings that are both trued and have that great Savarez sound. Any suggestions?

If you like the types of problems discussed in this page, please consider being a participant in the LEFTBRAINLUTHERIE mailing-list.  

    The list is now being managed by Australian luthier Jeremy Locke and is available at:

            leftbrainluthiers@yahoogroups.com

To explore more of the website, please refer to the Sitemap and Search Engine